Compensation Accessory Plates and Wedges

Compensation Accessory Plates and Wedges - Java Tutorial

A careful examination of anisotropy as a function of specimen orientation permits identification of the refractive index difference and orientation of the extraordinary and ordinary light rays produced by birefringent materials. This tutorial explores how compensators may be employed to help determine orientation parameters of anisotropic materials.

To rotate the tutorial, click and drag anywhere in the applet window.

In order to determine fast and slow light ray orientations in a birefringent material, the specimen is usually placed on a 360-degree rotating circular stage between crossed polarizers in an optical microscope. The axes of the specimen index ellipse are oriented diagonally, at a position corresponding to 45 degrees with respect to the vibration directions of the polarizer and analyzer. Next, a birefringent accessory or compensation plate composed of quartz, muscovite, or gypsum mounted in a specialized holder is added to the light path in a specific orientation. Compensation plates, which produce a fixed optical path difference between the specimen and plate, are mounted and carefully oriented in a rectangular frame so that the identities of the fast and slow vibration axes are known and fixed. The most common compensation plate is often referred to as a gypsum plate or full-wave plate, and has an optical path difference ranging between 550 and 580 nanometers.

As a rule, the slow ray (having a larger effective refractive index) is oriented in the Northwest-Southeast direction with respect to the polarizer and analyzer. Insertion of the first-order compensation plate (either before the specimen but after the polarizer or after the specimen but before the analyzer) to the light path will add the optical path difference (550 to 580 nanometers) to all optical paths in the microscope viewfield. The background, which is totally dark when an optical path difference of zero prevails (as is the case in crossed polarizers without a compensation plate), now appears magenta or red color, which is often termed Red I or first-order red. If the slow ray emanating from the specimen has a vibration vector that is parallel to the slow ray of the full-wave plate, the wavelength differences will add and a higher polarization color will appear. This effect is termed additive. In cases where the direction of the specimen and the full-wave plate slow rays are oriented at 90-degree angles to each other, wavelength differences will subtract and a lower polarization color will result (known as a subtractive effect). Rotation of the specimen by 90-degrees on the circular stage should confirm the identification of fast and slow rays.

As discussed above, the construction of most compensation plates assures that the long dimension of the plate is oriented Northwest-Southeast with respect to the microscope polarizer and analyzer vibration directions. The fast ray vibration direction is most often placed parallel to the compensation plate frame long axis (length), resulting in the slow ray vibration direction being oriented across the width or short axis. Therefore, when the compensation plate is placed between crossed polarizers, the fast ray vibration direction is Northwest-Southeast, while that of the slow ray is Northeast-Southwest. When a full-wave compensation plate is placed between the polarizers, the distinctive magenta color observed lies at the boundary between first and second order retardation colors. Other plates, such as a mica or gypsum plate, will produce lesser or varying degrees of retardation, depending upon the plate design. The mica plate yields an optical path difference of 140-155 nanometers (depending upon the manufacturer), while the quartz wedge produces a wide range of retardation values.

Compensators vary with respect to the range of optical path differences (number of orders) covered and how the birefringent element is placed in strategic orientations to permit introduction of continuously variable phase differences. Two primary factors determine the proper choice of compensator. A satisfactory compensator must be capable of compensating the largest expected optical path difference, and the sensitivity should be adequate to determine very small phase shifts. For instance, if an unknown birefringent crystal is suspected to have refractive index difference (n(e) - n(o)) around 0.25, and the thickness is approximately 20 microns, then the expected optical path difference is close to 500 nanometers. In this case, a first-order compensation plate (retardation of 550 nanometers) will adequately perform the task. Alternatively, a compensator with a range of four orders would have a very small adjustment with this specimen, leading to a less precise measurement.

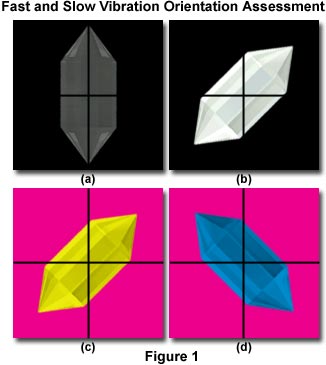
The following procedure is suggested for determining the identities of the vibration orientations for the slow and fast rays in an unknown birefringent material. First, rotate the microscope stage until the crystal displays the maximum amount of extinction (Figure 1(a)). The example crystal illustrated in Figure 1 has the slow ray vibration direction parallel to the long axis of the crystal and a corresponding fast ray vibration direction parallel to the short axis. By convention, the vibration direction of the polarizer is set to the East-West (abbreviated E-W position), while the analyzer is oriented with the vibration direction in the North-South (abbreviated N-S) orientation, at a 90-degree angle to the vibration direction of the polarizer. When the stage is rotated to orient the crystal at a 45-degree clockwise angle (with the long axis oriented Northeast-Southwest; Figure 1(b)), the maximum brightness is observed upon examination of the crystal through the microscope eyepieces. The crystal in Figure 1 appears white, but other crystals with a greater degree of thickness or birefringence can have first (or higher order) colors that can be deduced by examining a Michel-Levy color chart.

The next step is to insert a full-wave compensator into the microscope accessory slot to produce a 550-nanometer optical path difference, as illustrated in Figure 1(c). In order to determine whether the resulting interference color (yellow in Figure 1(c)) is higher or lower, an inspection is conducted and the results compared to a Michel-Levy color chart. Because the compensation plate adds 540 nanometers to all optical paths, interference colors produced by the specimen that are lower than this value represent a negative birefringence, while the higher order colors correspond to positive birefringence. In Figures 1(c) and 1(d), the interference colors are yellow (approximately 350 nanometers) and blue (approximately 650 nanometers), respectively. As previously discussed, the slow axis of the compensation plate is oriented perpendicular to the frame (Northeast-Southwest). Therefore, interference color wavelengths should be shifted to higher values when the slow axis of the compensator aligns with the slow axis of the crystal. Note that the crystal in Figure 1(c) exhibits an interference color of 350 nanometers (yellow), which indicates that the slow axis of the crystal is superimposed on the fast axis of the compensator, and perpendicular to the slow axis.

When the crystal is rotated 90-degrees in a counterclockwise direction (Figure 1(d)), higher order (second order blue) interference colors are seen through the microscope eyepieces. In this orientation, the slow axis of the crystal is parallel to the compensator slow axis. The evidence confirms that the fast ray refracted by the crystal has an axis that coincides with the long crystal axis and the slow ray is parallel to the crystal short axis. In general, interference color decreases are indicative of the slow crystal ray being parallel to the fast axis of the compensation. Likewise, interference color increases confirm that the crystal slow ray is parallel to the compensator slow axis.

If an unknown mineral exhibits a gray or first-order white interference color (approximately 200 nanometers), the color added by a first-order red plate yields a second-order color, while color subtraction results in a longer wavelength first-order color. In this case, a higher-order color is produced in both orientations.